khan academy greatest integer function
The greatest integer function takes an input and produces the greatest integer less than the input. First consider f x x if x is an integer then the value of f will be x itself.
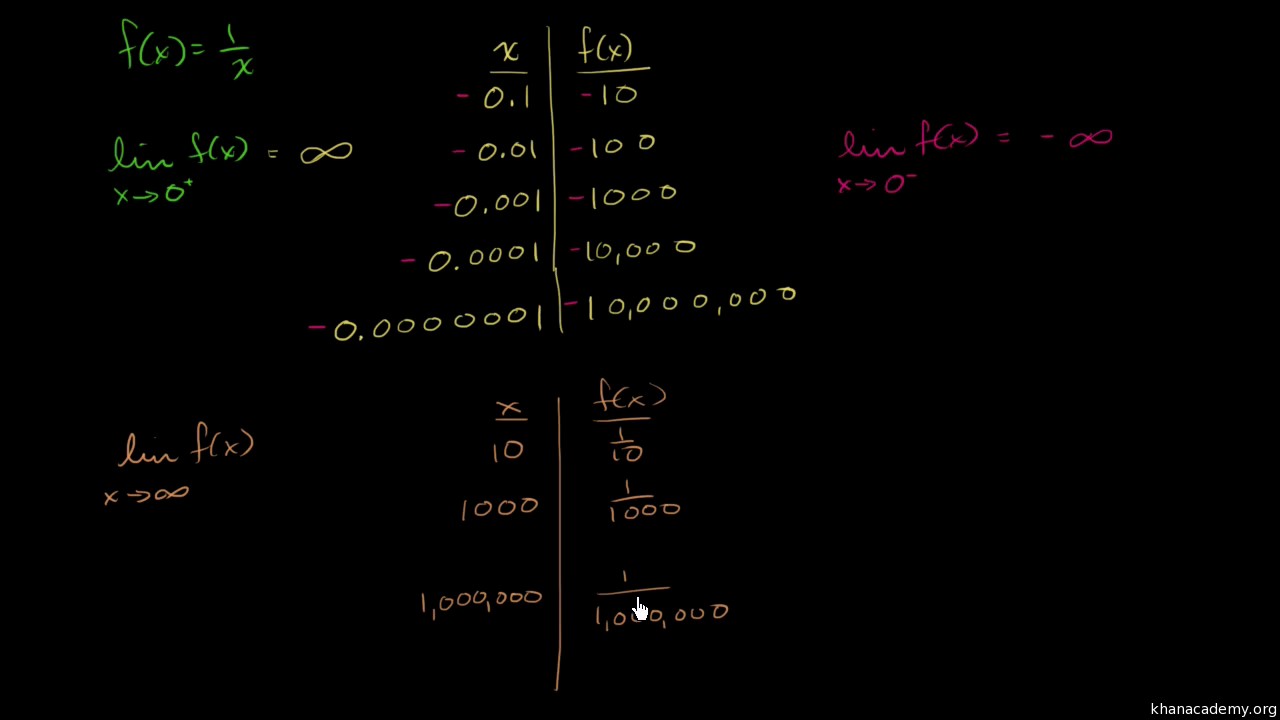
Infinite Limits Intro Video Khan Academy
Calculate the limits lim_.
. This video contains plenty of e. The graph is not continuous. Up to 10 cash back Correct answer.
For example the greatest integer function of the interval 34 will be 3. Opens a modal Worked example. For example consider the number 115.
If. Opens a modal Worked example. This video concerns itself with the properties and characteristics of the Greatest Integer Function its equation and its graph.
One of the most commonly used step functions is the greatest integer function. If the input is an integer then the output is that integer. Whole numbers integers.
The greatest integer function also known as the floor function is defined by left lfloor x right rfloor n where n is the unique integer n leq x lt n1. What is a function. This function has a step curve and is also recognized as the step function.
In essence it rounds down to the the nearest integer. Thus the output is always smaller than the input and is an integer itself. Y x For all real values of x the greatest integer function returns the greatest integer which is less than or equal to x.
Algebra Applied Mathematics Calculus and Analysis Discrete Mathematics Foundations of Mathematics Geometry History and Terminology Number Theory Probability and Statistics Recreational Mathematics Topology Alphabetical Index New in. Greatest integer function graph. For example is the number -8 a whole number.
The table shows us that the function increases to the next highest integer any time the x-value becomes an integer. The greatest integer function takes an input and the output is given based on the following two rules. For the entire interval 12 f will take.
Evaluating functions from graph. Ordering negative numbers Get 5 of 7 questions to level up. Khan Academy is a 501c3 nonprofit organization.
For instance below is the graph of the function f x x. According to the greatest integer function 115 1. The greatest integer function denoted by x is any real function that rounds off a real number down to an integer less than that number.
If x is a non-integer then the value of x will be the integer just before x. Is it an integer. For any real number x let brackets around x denote the largest integer less than or equal to x often known as the greatest integer function.
The greatest integer function is denoted by. The function which produces the greatest integer less than or equal to the number operated upon. Learn complete concept of Greatest Integer Function which also called Floor function or step function in Relations and Function Mathematics.
For example For all numbers lying in the interval 01 the value of f will be 0. Xthe largest integer that is less than or equal to x. When the intervals are in the form of n n1 the value of greatest integer function is n where n is an integer.
Walk through the difference between whole numbers integers. First well learn about whole numbers then well learn about integers and well finish by thinking about. Opens a modal Worked example.
This calculus video tutorial explains how to graph the greatest integer function and how to evaluate limits that contain it. Evaluating functions from equation. The domain and range of the greatest integer function is R and Z respectively.
Similarly the greatest integer of 456 is 456 4. Sketch a graph of y 1 2 x. The graph of the greatest integer function resembles an ascending.
Opens a modal Evaluating discrete functions. It is also known as the floor of X. The greatest Integer Function X indicates an integral part of the real number which is the nearest and smaller integer to.
This results in the following graph. The greatest integer function rounds up the number to the most neighboring integer less than or equal to the provided number. Let us plot the greatest integer function graph.
Graphing piecewise functions Our mission is to provide a free world-class education to anyone anywhere. The greatest integer function has its own notation and tells us to round whatever decimal number it is given down to the nearest integer or the greatest integer that is less than the number. It is also known as the floor of X.
Let f be a real valued function defined on the interval negative 10 to 10 including the boundaries by f of x is equal to x minus the greatest integer of x if the greatest integer of x is odd and 1. We also call this function a Floor Function. Since our input was we are looking for an integer less than this which must be since any smaller integer would by definition not be greatest.
We know what the basic graph should look like so we just need to understand how the factor of 1 2 is going to affect things. Negative numbers variables number line Get 3 of 4 questions to level up.

Transformation And The Greatest Integer Function Youtube

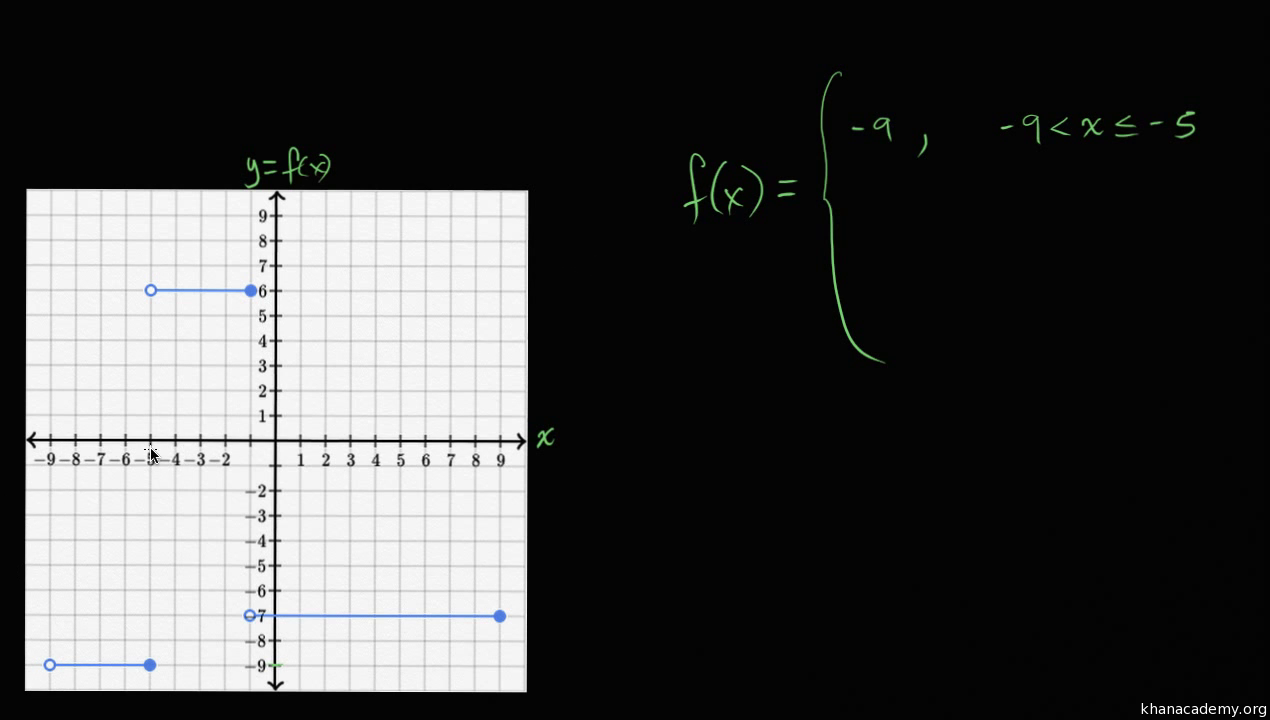
Worked Example Evaluating Piecewise Functions Video Khan Academy

Transformation And The Greatest Integer Function Youtube
Comparing Linear Functions Faster Rate Of Change Video Khan Academy

Ex Limits Involving The Greatest Integer Function Youtube
Introduction To Piecewise Functions Algebra Video Khan Academy

The Greatest Integer Function Youtube

Graph F X 3x The Greatest Integer Function Youtube

Graphing Calculator Greatest Integer Function Youtube
Operations And Algebra 227 228 Map Recommended Practice Khan Academy
Functions Algebra All Content Math Khan Academy

Introduction To Piecewise Functions Algebra Video Khan Academy
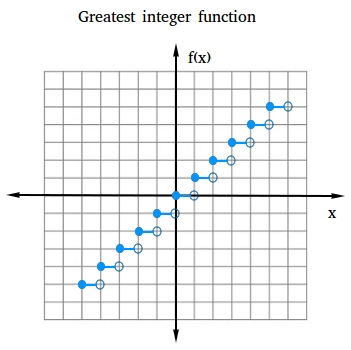
Greatest Integer Function Definition Examples And Graph
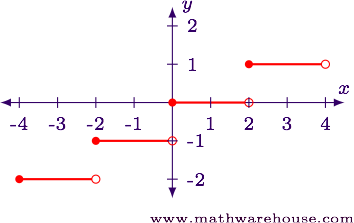
Greatest Integer Function And Graph

Limits Of Trigonometric Functions Video Khan Academy
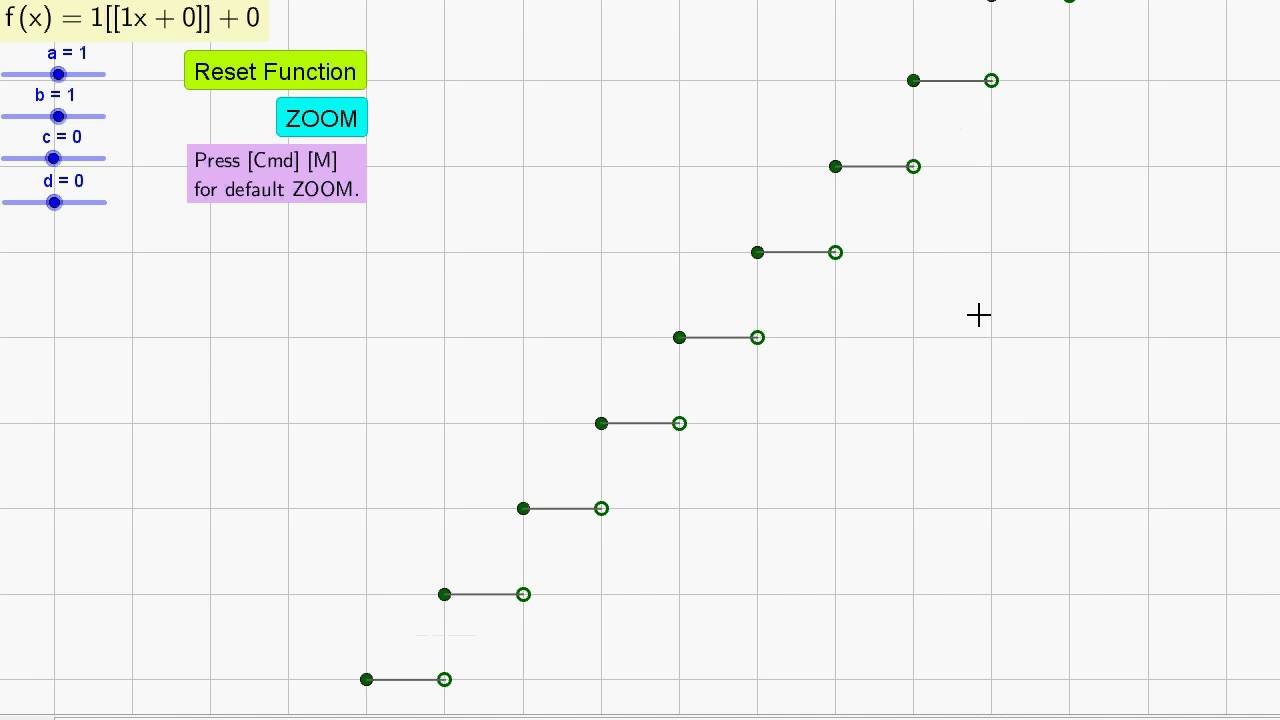
Best Geogebra Function Graphs 14 Greatest Integer Function With Infinite Number Of Holes Youtube
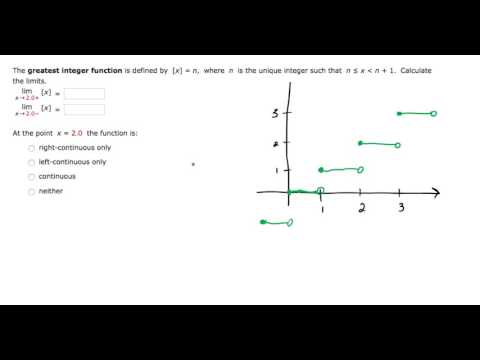
Continuity And The Greatest Integer Function Youtube

Greatest Integer Function With Limits Graphs Youtube

Greatest Integer Function Floor Function Step Function In Mathematics Youtube